The compilation of these Index Numbers Notes makes students exam preparation simpler and organised.
Index Numbers in General
India ranks 46th on the quality of life index with Denmark sitting at the top. Did you know how such ranks are calculated? Our country’s rank is as much of a concern as index numbers are because such calculations are done using index numbers. Let us dive deep into the sea of index numbers.
Index Numbers

So what are index numbers? Well, technically speaking, an index number is a statistical measure designed to show changes in a variable or group of related variables with respect to time, geographic location or other characteristics.
Let’s understand this with an example. Suppose the price of a certain product doubles relative to a year. As a result of this, the index of price would rise. So an index number can be with respect to a variable such as a price and demonstrates the change in that variable in present relative to a base year.
An index number is not an absolute measure, it measures the percentage change in a variable over time. It does so by comparing the value of a variable at present to its value at a base year. Index number gives a quantitative foundation to qualitative statements like prices are falling or rising. Lastly, index numbers show changes in average. In effect, it means if the average change is 5% then some goods might not change exactly at 5%.
Why Use Index Numbers?
The most important use of index numbers is the determination of the value of money using price index numbers. It effectively displays the change in price levels and depicts inflation or deflation. As already mentioned index numbers are used to calculate the standard of living in various areas. Price index numbers are extensively used by business communities as a tool to plan policies.
To calculate the change in the production of various sectors, index numbers of production are used. This, in turn, helps the government to take necessary measures to increase production and growth. Export and import policies are formulated by referring to the index of imports and exports. Lastly, the government plans its fiscal and monetary policies to increase growth, employment, productivity, etc. with the aid of index numbers. By and large, an index number is an important statistical tool.
The Negative Side
Index numbers deal with averages and are a rough estimate of the change in a variable. Consequently, they simply indicate temporal changes for the variable which are not completely true or accurate. Also with changing times, consumptions patterns undergo a drastic change. As a result, a comparison of previous index numbers to recent ones doesn’t seem viable.
Calculation of retail price index number is not possible hence we calculate wholesale price index number although the former one displays the real scenario. Lastly, for each variable, we have to calculate different index numbers, which widens our calculations.
Simple Index Numbers
Before moving forward with the construction of an index number, we shall discuss the two broad classifications of these numbers.
Simple index numbers grant equal importance to all items no matter what share it has. In other words, it considers each item to be equal with respect to the given variable. Consequently, it is a simple average and is less accurate when compared to the other class of index numbers.
Weighted Index Numbers
Unlike simple index numbers, weighted index numbers, as the name suggests, weigh items according to their importance with respect to the concerned variable. For example, when calculating the price index number if the price of a unit of rice is twice the price of a unit of sugar then the rice will be weighed in as ‘2’ whereas sugar will be weighed in as ‘1’.
Hence it is a relatively average measure. In essence, it is more realistic in comparison to a simple index numbers because it accurately reflects the change over time.
Construction of Index Numbers
- Current year: It refers to the year for which we aim to find the index number.
- Base year: It acts as the reference for which we wish to find the change in the value of the variable.
There are two methods of deducing formulae for each of the two types of index numbers.
Average or Price Relatives Method
Aggregative Method
Construction of Simple Index Numbers
1. Simple Average or Price Relatives Method
In this method, we find out the price relative to individual items and average out the individual values. Price relative refers to the percentage ratio of the value of a variable in the current year to its value in the year chosen as the base.
Price relative (R) = (P1 ÷ P2) × 100
Here, P1 = Current year value of the item with respect to the variable and
P2 = Base year value of the item with respect to the variable.
Effectively, the formula for index number according to this method is:
P = ∑[(P1 ÷ P2) × 100] ÷ N
Here, N = Number of goods and
P = Index number.
2. Simple Aggregative Method
It calculates the percentage ratio between the aggregate prices of all commodities in the current year and the aggregate prices of all commodities in the base year.
P = (∑P1 ÷ ∑P2) × 100
Here, ∑P1 = Summation of the prices of all commodities in the current year and
∑P2 = Summation of prices of all commodities in the base year.
Construction of Weighted Index Number
1. Weighted Average or Price Relatives Method
Here we calculate the ratio between the summation of the product of weights with price relatives and the summation of the weights.
P = ∑RW ÷ ∑W
Here, R= Price relative and
W= weight.
2. Weighted Aggregate Method
Here different goods are assigned a weight according to the quantity bought. There are three well-known sub-methods based on the different views of economists as mentioned below:
A. Laspeyre’s Method
Laspeyres was of the view that base year quantities must be chosen as weights. Therefore the formula is:
P = (∑P1Q0 ÷ ∑P0Q0) × 100
Here, ∑P1Q0 = Summation of prices of the current year multiplied by quantities of the base year taken as weights and
∑P0Q0 = Summation of, prices of the base year multiplied by quantities of the base year taken as weights.
B. Paasche’s Method
Unlike the above mentioned, Paasche believed that the quantities of the current year must be taken as weights. Hence the formula:
P = (∑P1Q1 ÷ ∑P0Q1) × 100
Here, ∑P1Q1 = Summation of, prices of the current year multiplied by quantities of the current year taken as weights and
∑P0Q1 = Summation of, prices of the base year multiplied with quantities of the current year taken as weights.
C. Fisher’s Method
Fisher combined the best of both above-mentioned formulas which resulted in an ideal method. This method uses both current and base year quantities as weights as follows:
P = √[(∑P1Q0 ÷ ∑P0Q0) × (∑P1Q1 ÷ ∑P0Q1)] × 100
Note: The index number of the base year is generally assumed to be 100 if not given
Fisher’s Method is an Ideal Measure
As noted Fisher’s method uses views of both Laspeyres and Paasche. Hence it takes into account the prices and quantities of both years. Moreover, it is based on the concept of the geometric mean, which is considered the best mean method.
However, the most important evidence for the above affirmation is that it satisfies both time-reversal and factor reversal tests. Time reversal test checks that when we reverse the current year to the base year and vice-versa, the product of indexes should be equal to unity. This confirms the working of the formula in both directions. Also, the factor reversal test implies that interchanging the piece and quantities does not give varying results. This proves the consistency of the formula.
Common Problems with Construction of Index Numbers
Due to the availability of a wide range of index numbers we have to select an index number that matches the objective we want to fulfill. For example, to study the impact of a change in the government’s budget on people, one should refer to the price index number.
It must be noted that the selected base year should be a normal one. In other words, there should be no reforms in that year that can influence the economy in a drastic manner. If such is chosen as the base year there will be a big variation in the index numbers, which would not reflect the accurate changes over the years.
Also, it is not possible to include all the goods and services along with their prices in our calculations. This means we need to select various goods and services that can effectively represent all of them. In a word, the sample size has to be selected. The larger the sample size more is the accuracy. And we need to select the method of calculation that suits best with the objective in hand.
Example:
Question:
Construct index numbers of prices of items in the year 2012 from the following data by:
1. Laspeyres method
2. Paasche’s method
3. Fisher’s method

Answer:
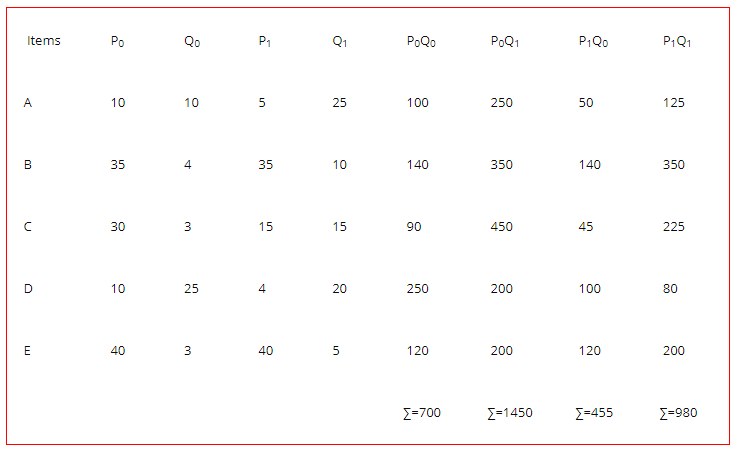
Laspeyre’s method = (455/700) × 100 = 65
Paasche’s method = (980/1450) × 100 = 67.58
Fisher’s method = √0.43927 × 100 = 66.27