Maharashtra Board Class 10 Maths Solutions Chapter 1 Linear Equations in Two Variables Practice Set 1.3

Maharashtra state Board 10th Standard Solutions Chapter 1 Linear Equations in two Variables – Here are all the MH Board solutions for 10th Standard maths Practice Set 1.3. This solution contains questions, answers, images, explanations of the complete Practice Set 1.3 titled Linear Equations in two Variables of maths taught in 10th Standard. If you are a student of 10th Standard who is using Maharashtra state Board Textbook to study maths, then you must come across Practice Set 1.3 Linear Equations in two Variables. After you have studied lesson, you must be looking for answers of its questions. Here you can get complete Maharashtra Board Solutions for 10th Standard maths Chapter 1 Linear Equations in two Variables in one place.

Maharashtra State Board Class 10 Maths Solutions Chapter 1 Linear Equations in Two Variables Practice Set 1.3

Question 1.
Fill in the blanks with correct number.
Solution:

Question 2.
Find the values of following determinants.

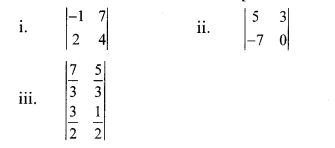
Solution:

Question 3.
Solve the following simultaneous equations using Cramer’s rule.
i. 3x – 4y = 10 ; 4x + 3y = 5
ii. 4x + 3y – 4 = 0 ; 6x = 8 – 5y
iii. x + 2y = -1 ; 2x – 3y = 12
iv. 6x – 4y = -12 ; 8x – 3y = -2
v. 4m + 6n = 54 ; 3m + 2n = 28
vi. 2x + 3y = 2 ; x – \(\frac { y }{ 2 } \) = \(\frac { 1 }{ 2 } \)
Solution:
i. The given simultaneous equations are 3x – 4y = 10 …(i)
4x + 3y = 5 …(ii)
Equations (i) and (ii) are in ax + by = c form.
Comparing the given equations with
a₁x + b₁y = c₁ and a₂x + b₂y = c₂, we get
a₁ = 3, b₁ = -4, c₁ = 10 and
a₂ = 4, b₂ = 3, c₂ = 5

∴ (x, y) = (2, -1) is the solution of the given simultaneous equations.

ii. The given simultaneous equations are
4x + 3y – 4 = 0
∴ 4x + 3y = 4 …(i)
6x = 8 – 5y
∴ 6x + 5y = 8 …(ii)
Equations (i) and (ii) are in ax + by = c form.
Comparing the given equations with
a₁x + b₁y = c₁ and a₂x + b₂y = c₂, we get
a₁ = 4, b₁ = 3, c₁ = 4 and
a₂ = 6, b₂ = 5, c₂ = 8

∴ (x, y) = (-2, 4) is the solution of the given simultaneous equations.

iii. The given simultaneous equations are
x + 2y = -1 …(i)
2x – 3y = 12 …(ii)
Equations (i) and (ii) are in ax + by = c form.
Comparing the given equations with
a₁x + b₁y = C₁ and a₂x + b₂y = c₂, we get
a₁ = 1, b₁ = 2, c₁ = -1 and
a₂ = 2, b₂ = -3, c₂ = 12

∴ (x, y) = (3, -2) is the solution of the given simultaneous equations.

iv. The given simultaneous equations are
6x – 4y = -12
∴ 3x – 2y = -6 …(i) [Dividing both sides by 2]
8x – 3y = -2 …(ii)
Equations (i) and (ii) are in ax + by = c form.
Comparing the given equations with
a₁x + b₁y = c₁ and a₂x + b₂y = c₂, we get
a₁ = 3, b₁ = -2, c₁ = -6 and
a₂ = 8, b₂ = -3, c₂ = -2

∴ (x, y) = (2, 6) is the solution of the given simultaneous equations.

v. The given simultaneous equations are
4m + 6n = 54
2m + 3n = 27 …(i) [Dividing both sides by 2]
3m + 2n = 28 …(ii)
Equations (i) and (ii) are in am + bn = c form.
Comparing the given equations with
a₁m + b₁n = c₁ and a₂m + b₂n = c₂, we get
a₁ = 2, b₁ = 3, c₁ = 27 and
a₂ = 3, b₂ = 2, c₂ = 28


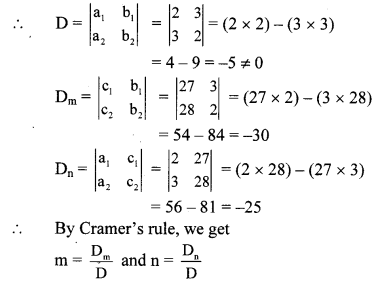
∴ (m, n) = (6, 5) is the solution of the given simultaneous equations.

vi. The given simultaneous equations are
2x + 3y = 2 …(i)
x = \(\frac { y }{ 2 } \) = \(\frac { 1 }{ 2 } \)
∴ 2x – y = 1 …(ii) [Multiplying both sides by 2]
Equations (i) and (ii) are in ax + by = c form.
Comparing the given equations with
a₁x + b₁y = c₁ and a₂x + b₂y = c₂, we get
a₁ = 2, b₁ = 3, c₁ = 2 and
a₂ = 2, b₂ = -1, c₂ = 1

Question 1.
To solve the simultaneous equations by determinant method, fill in the blanks,
y + 2x – 19 = 0; 2x – 3y + 3 = 0 (Textbookpg.no. 14)
Solution:
Write the given equations in the form
ax + by = c.
2x + y = 19
2x – 3y = -3

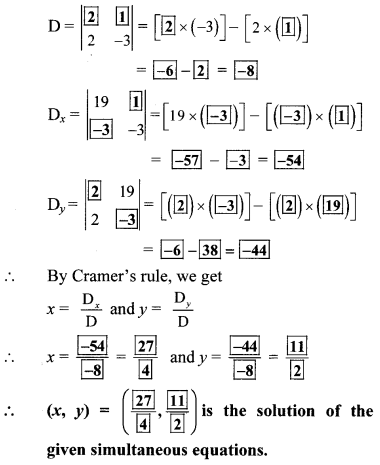
Question 2.
Complete the following activity. (Textbook pg. no. 15)
Solution:

Question 3.
What is the nature of solution if D = 0? (Textbook pg. no. 16)
Solution:
If D = 0, i.e. a₁b₂ – b₁a₂ = 0, then the two simultaneous equations do not have a unique solution.
Examples:
i. 2x – 4y = 8 and x – 2y = 4
Here, a₁b₂ – b₁a₂ = (2)(-2) – (-4) (1)
= -4 + 4 = 0
Graphically, we can check that these two lines coincide and hence will have infinite solutions.

ii. 2x – y = -1 and 2x – y = -4
Here, a₁ b₂ – b₁ a₂ = (2)(-1) – (-1) (2)
= -2 + 2 = 0
Graphically, we can check that these two lines are parallel and hence they do not have a solution.

Question 4.
What can you say about lines if common solution is not possible? (Textbook pg. no. 16)
Answer:
If the common solution is not possible, then the lines will either coincide or will be parallel to each other.

Maharashtra Board Class 10 Maths Solutions